Integration

Understanding Double Integrals: One Step at a Time

Jake

Oct 10, 2023 • 5 min read

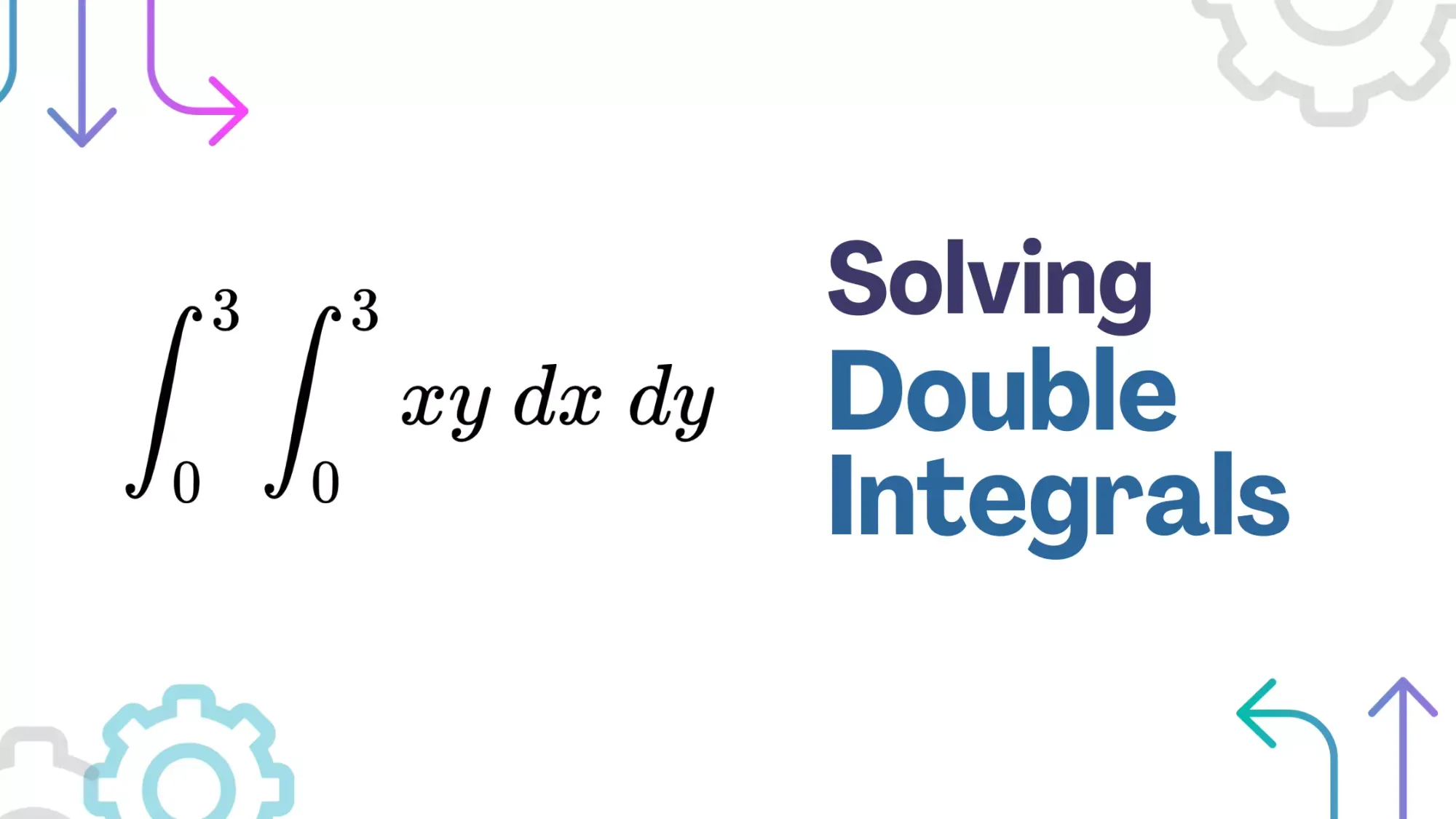
Hey there math lovers! 📚✏️ Ever been out and about, perhaps sipping on your morning coffee, when someone casually mentions "double integrals" and you think, "What in the world is that?" Or maybe you've encountered them in a math class, and they seemed like a daunting mountain to climb. Either way, you're in the right place!

Today, we're embarking on an exciting journey into the realm of double integrals. If you've ever been curious about how mathematicians calculate the volume beneath those wavy, curvy surfaces, you're about to unlock that secret. And guess what? It's not as complicated as it sounds! By the end of this post, not only will you understand the concepts, but you'll also be able to solve them with confidence. So, whether you're a curious cat, a budding mathematician, or just someone who loves a good math challenge, let's unravel this mystery together!

Imagine you're trying to find the volume under a surface. Single integrals are like calculating the area under a curve, but when we venture into the 3D world, we need double integrals. Think of it as trying to find out how much water is in a wavy bathtub - not by filling it, but by mathematically calculating every tiny slice of volume.

What's a Double Integral

Double integrals may sound like a complex concept, but at their heart, they're a natural extension of what you're familiar with—simple integrals. Recall how regular integrals compute the area beneath a curve. Now, elevate that thinking to 3D space. Instead of a curve, imagine a surface soaring above the ground, with peaks and valleys. Double integrals come to the rescue, helping us calculate the volume underneath this surface. It's like summing up the tiny columns or pillars holding up that surface.

This technique isn't just about volumes, though. Depending on the context, double integrals can represent various accumulated quantities, from densities to masses. While the underlying math can get intricate, especially when we venture beyond simple rectangular regions, the foundational idea remains consistent: slice up the area into manageable bits, then sum them up. As with many mathematical concepts, practice and understanding the basics pave the way for mastering more complex scenarios.

Setting the Stage: The Basic Notation

For a function \(f(x,y)\) defined over a region \(R\), the double integral is denoted as:

\[
\int \int_R f(x,y) \, dA
\]

Where \(dA\) is a tiny area element in the \(xy\)-plane.

The Process: Breaking It Down

Step 1: Choose the Order of Integration

Decide if you're integrating with respect to \(x\) or \(y\) first. This can change the complexity of the integral!

Example:
Given the function \(f(x,y) = xy\), and the region \(R\) is the rectangle defined by \(0 \leq x \leq 2\) and \(0 \leq y \leq 3\).

The double integral can be set up in two ways:

Integrate with respect to \(x\) first, then \(y\):
\[
\int_0^3 \int_0^2 xy \, dx \, dy
\]
Integrate with respect to \(y\) first, then \(x\):
\[
\int_0^2 \int_0^3 xy \, dy \, dx
\]

Step 2: Evaluate the Inner Integral

Treat the outer variable as a constant and integrate it with respect to the inner variable.

Example (using the first order from above):

Inner Integral:
\[
\int_0^2 xy \, dx = \frac{x^2y}{2} \Bigg|_0^2 = 2y
\]

Step 3: Evaluate the Outer Integral

Now, take the result from the inner integral and integrate it with respect to the outer variable.

Example (continuing from above):

Outer Integral:
\[
\int_0^3 2y \, dy = y^2 \Bigg|_0^3 = 9
\]

Elementary Examples of Double Integrals

Let's break down a few basic examples of solving double integrals. For clarity, I'll be using rectangular regions, but remember that double integrals can be applied over more complex regions as well.

Example 1: Constant Function

Function: \( f(x,y) = 5 \) over the rectangle \( 0 \leq x \leq 2 \) and \( 1 \leq y \leq 3 \).

Step 1: Set up the double integral. \[ \int_1^3 \int_0^2 5 \, dx \, dy \]
Step 2: Integrate with respect to \(x\). \[ \int_0^2 5 \, dx = 5x \Bigg|_0^2 = 10 \]
Step 3: Integrate the result with respect to \(y\). \[ \int_1^3 10 \, dy = 10y \Bigg|_1^3 = 20 \]

Final Result: \( 20 \)

Example 2: Linear Function in x and y

Function: \( f(x,y) = x + y \) over the rectangle \( 0 \leq x \leq 3 \) and \( 0 \leq y \leq 4 \).

Step 1: Set up the double integral. \[ \int_0^4 \int_0^3 (x + y) \, dx \, dy \]
Step 2: Integrate with respect to \(x\). \[ \int_0^3 (x + y) \, dx = \left(\frac{x^2}{2} + xy\right) \Bigg|_0^3 = \frac{9}{2} + 3y \]
Step 3: Integrate the result with respect to \(y\). \[ \int_0^4 \left(\frac{9}{2} + 3y\right) \, dy = \left(\frac{9}{2}y + \frac{3y^2}{2}\right) \Bigg|_0^4 = 36 \]

Final Result: \( 36 \)

Example 3: Quadratic Function

Function: \( f(x,y) = x^2 + y^2 \) over the rectangle \( 0 \leq x \leq 1 \) and \( 0 \leq y \leq 2 \).

Step 1: Set up the double integral. \[ \int_0^2 \int_0^1 (x^2 + y^2) \, dx \, dy \]
Step 2: Integrate with respect to \(x\). \[ \int_0^1 (x^2 + y^2) \, dx = \left(\frac{x^3}{3} + xy^2\right) \Bigg|_0^1 = \frac{1}{3} + y^2
\]
Step 3: Integrate the result with respect to \(y\). \[ \int_0^2 \left(\frac{1}{3} + y^2\right) , dy = \left(\frac{y}{3} + \frac{y^3}{3}\right) \Bigg|_0^2 = \frac{8}{3} + \frac{2}{3} = \frac{10}{3} \]

Final Result: \( \frac{10}{3} \)

These step-by-step solutions demonstrate the sequential process of evaluating double integrals. Each inner integral is evaluated first, and then its result is integrated with respect to the next variable.

Regions Aren't Always Rectangles

Sometimes, the region \(R\) isn't a simple rectangle. This is where the fun (and the challenge!) begins. For non-rectangular regions, the limits of integration aren't constants but can be functions of \(x\) or \(y\).

Example:
Find the double integral of \(f(x,y) = x^2y\) over the triangular region defined by the vertices (0,0), (2,0), and (2,2).

The region can be described by:

\(0 \leq x \leq 2\)
\(0 \leq y \leq x\)

The double integral is:
\[
\int_0^2 \int_0^x x^2y \, dy \, dx
\]

Changing to Polar Coordinates

Sometimes, it's easier to solve a double integral using polar coordinates, especially when dealing with circles or radial symmetry.

Example:
Find the double integral of \(f(r,\theta) = r^2\) over the circle of radius 2 centered at the origin.

The integral in polar coordinates is:
\[
\int_0^{2\pi} \int_0^2 r^2 \cdot r \, dr \, d\theta
\]
Note the extra \(r\) - it’s the Jacobian for the change of variables!

Wrapping It Up

So, we've journeyed through the intriguing world of double integrals, taking them apart and piecing them together, step-by-step. It's fascinating, isn't it? How these mathematical constructs can help us visualize and calculate volumes beneath complex surfaces. If you've stuck with me till now, give yourself a pat on the back! 🎉

Remember, like any skill, mastering double integrals takes practice. So don't be disheartened if they seem tricky at first. With each problem you tackle, you'll become more familiar with the process, and soon you'll be integrating with ease.

Now, as you move forward and encounter other mathematical challenges, don't forget the essence of this lesson: Every complex topic becomes simpler when broken down. And, as always, the world of math is full of surprises and wonders waiting to be explored. So, keep those pencils sharpened, those minds open, and never stop being curious. Who knows? Maybe the next time you're at that coffee shop and someone mentions "double integrals," you'll be the one enlightening them!

Until our next math adventure, keep integrating, keep learning, and above all, enjoy the journey. After all, math isn't just about finding the right answers, but also cherishing the beauty of the questions.